Finding optimal rotation and translation between corresponding 3. D points. Last update 1. May 2. 01. 3Fixed a mistake in handling reflection case. Finding the optimalbest rotation and translation between two sets of corresponding 3. D point data, so that they are alignedregistered, is a common problem I come across. Play Store For Samsung Wave Y Bada. An  illustration of the problem is shown below for the simplest case of 3 corresponding points the minimum required points to solve. Python program for reversal algorithm of array rotation Function to reverse arr from index start to end def rverseArrayarr, start, end while start lt end. The corresponding points have the same colour, R is the rotation and t is the translation. We want to find the best rotation and translation that will align the points in dataset A to dataset B. Here, optimal or best is in terms of least square errors. This transformation is sometimes called the Euclidean or Rigid transform, because it preserves the shape and size. This is in contrast to an affine transform, which includes scaling and shearing. This problem arises especially in tasks like 3. D point cloud data registration, where the data is obtained from hardware like a 3. D laser scanner or the popular Kinect device. The solution Ill be presenting is from the paper A Method for Registration of 3 D Shapes, by Besl and Mc. UxtojbMsAzs/hqdefault.jpg' alt='Matrix Rotation Program In C' title='Matrix Rotation Program In C' />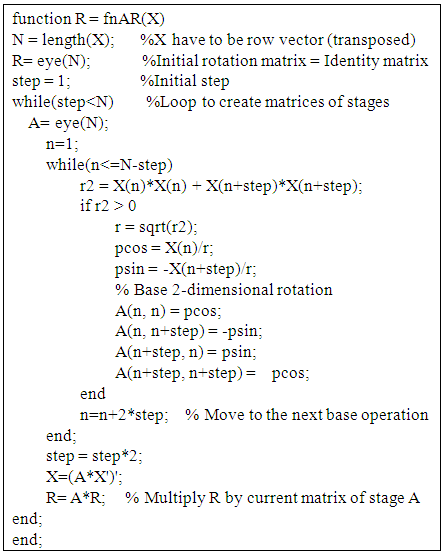Kay, 1. Solution overview. Were solving for R,t in the equation B RA t. Where R,t are the transforms applied to dataset A to align it with dataset B, as best as possible. Finding the optimal rigid transformation matrix can be broken down into the following steps Find the centroids of both dataset. Bring both dataset to the origin then find the optimal rotation, matrix RFind the translation t. Finding the centroids. This bit is easy, the centroids are just the average point and can be calculated as follows Here, and are points in dataset A and B respectively. We will use these values in the next step. Finding the optimal rotation. Finding optimal rotation and translation between corresponding 3D points. Instead of using a simple lifetime average, Udemy calculates a courses star rating by considering a number of different factors such as the number of ratings, the. Certified Unified Program Agency CUPA INSPECTION AND ENFORCEMENT PLAN Environmental Management Department EMD Environmental Compliance Division. Write a function rotatear, d, n that rotates arr of size n by d elements. Rotation of the above array by 2 will make array. There are a few ways of finding optimal rotations between points. The easiest way I found is using Singular Value Decomposition SVD, because its a function that is widely available in many programming languages Matlab, Octave, C using LAPACK, C using Open. CV. SVD is like this powerful magical wand in linear algebra for solving all sorts of numerical problems, but tragically wasnt taught when I was at uni. I wont go into details on how it works but rather how to use it. You only need to know that the SVD will decomposefactorise a matrix call it E, into 3 other matrices, such that If E is a square matrix then U, S and V are the same size as well. Rotation+about+a+Fixed+Point.jpg' alt='Matrix Rotation Program In C' title='Matrix Rotation Program In C' />Were only interested in square matrices for this problem so I wont go into detail about rectangular ones. To find the optimal rotation we first re centre both dataset so that both centroids are at the origin, like shown below. This removes the translation component, leaving on the rotation to deal with. The next step involves accumulating a matrix, called H, and using SVD to find the rotation as follows H is the familiar covariance matrix. At this point you may be thinking, what the, that easy, and indeed you would be right. One thing to be careful is that you calculate H correctly. It should end up being a 33 matrix, not a 11 matrix. Pay close attention to the transpose symbol. Its doing a multiplication between 2 matrices where the dimensions effectively are, 31 and 13, respectively. The ordering of the multiplication is also important, doing it the other way will find a rotation from B to A instead. Special reflection case. Theres a special case when finding the rotation matrix that you have to take care of. Sometimes the SVD will return a reflection matrix, which is numerically correct but is actually nonsense in real life. This is addressed by checking the determinant of R from SVD above and seeing if its negative 1. Download Pitbull The Boat Lift RARE'>Download Pitbull The Boat Lift RARE. If it is then the 3rd column of V is multiplied by 1. R lt 0. multiply 3rd column of V by 1. R. end if. An alternative check that is possibly more robust was suggested by Nick Lambertif determinantR lt 0. R by 1. end ifwhere R is the rotation matrix. A big thank you goes to Klass Jan Russcher and Nick Lambert for these solutions. Finding t. The translation is. The centroids are 31 column vectors. Adobe Photoshop Cs3 Crack Keygen. How did I get this If you remember back, to transform A to B we first had to centre A to its origin. This is where the centroidA comes from, though I put the minus sign at the front. We then rotate A, hence R. Then finally translate it to dataset Bs origin, the centroidB bit. And were done Note on usage. The solution presented can be used on any size dataset as long as there are at least 3 points. When there are more than 3 points a least square solution is obtained, such that the following error is minimised Here, the operator   is the Euclidean distance between two vectors, a scalar value. Code. This script has been tested in Octave. It should work in Matlab but it has not been tested. Ive also done a Python version still learning the language. Both scripts come with an example on how to use. D. mrigidtransform3. D. py rename the file to remove the trailing, added to stop the webserver from parsing itRead the comments in the Octave file for usage.